When producing a good or service, how do suppliers determine the quantity of factors to hire? Below, we work through an example where a representative producer answers this question.
Letís begin by making some assumptions. First, we shall assume that our producer chooses varying amounts of two factors, capital (K) and labor (L). Each factor was a price that does not vary with output. That is, the price of each unit of labor (w) and the price of each unit of capital (r) are assumed constant. Weíll further assume that w = $10 and r = $50. We can use this information to determine the producerís total cost. We call the total cost equation an isocost line (itís similar to a budget constraint).
The producerís isocost line is:
|
10L + 50K = TC |
(1) |
The producerís production function is assumed to take the following form:
|
q = (KL)0.5 |
(2) |
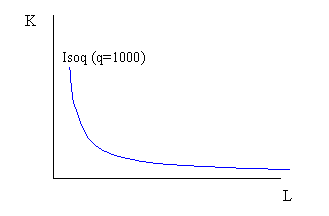
Our producerís first step is to decide how much output to produce. Suppose that quantity is 1000 units of output. In order to produce those 1000 units of output, our producer must get a combination of L and K that makes (2) equal to 1000. Implicitly, this means that we must find a particular isoquant.
Set (2) equal to 1000 units of output, and solve for K. Doing so, we get the following equation for a specific isoquant (one of many possible isoquants):
|
K = 1,000,000/L |
(2a) |
For any given value of L, (2a) gives us a corresponding value for K. Graphing these values, with K on the vertical axis and L on the horizontal axis, we obtain the blue line on the graph below. Each point on this curve is represented as a combination of K and L that yields an output level of 1000 units. Therefore, as we move along this isoquant output is constant (much like the fact that utility is constant as we move along an indifference curve).

How much K and L should the producer hire? The answer is that our choice must exist somewhere on this isoquant. If each possible choice must lie on this isoquant, then our basis for choosing one "best" combination should be to choose the least cost combination. Letís experiment with some different possibilities, by plugging values for L and K into the isocost equation above. Each combination should yield output of 1000 units. Several combinations, and their specific total cost are given as follows:
|
L |
K |
TC |
|
1,000 |
1,000 |
60,000 |
|
5,000 |
200 |
60,000 |
|
10,000 |
100 |
105,000 |
|
100 |
10,000 |
501,000 |
Now, the firmís goal is to produce 1000 units at the lowest possible TC. The lowest total cost on the table is $60,000, so we can start there. There are two choices which yield this total cost. They are represented below as B1 and B2.
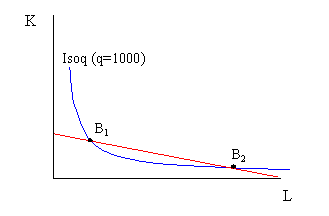
Is there a lower cost combination of L and K available? Yes, we can produce at a total cost of $52,500 by employing either 250 units of K and 4000 units of L, or 800 units of K and 1250 units of L. This appears on the graph below.
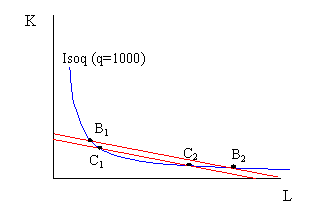
The second isocost, where TC = $52,500, rests below the former isocost, where TC = $60,000. If we continue to find lower and lower levels of total cost that provide us with 1000 units of output, then we will clearly reach lower and lower points on the isoquant. Eventually, we can find a level of total cost that involves a tangency between the isocost and isoquant. This is pictured below at point A.
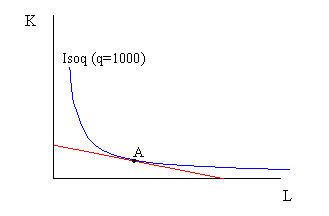
In addition to being the lowest cost combination of L and K that produces 1000 units of output, pt. A involves a tangency point between the isoquant and isocost. That is, the slopes of these curves at pt. A are equal. The slope of the isocost is w/r, or Ė1/5. The slope of the isoquant is the ratio of the marginal products, MPL/MPK, which is given as the marginal rate of technical substitution (MRTS). Using calculus, it is possible to derive the MRTS as ĖK/L. Point A satisfies the condition that K/L = 1/5.
We can solve for K* and L* at pt. A, using (1), (2a) and the fact that, at pt. A, K/L = 1/5. First, substitute (2a) into (1) and the equation K/L = 1/5. We're left with:
Solve the second equation for L, substitute that result into the first equation to get the lowest value for TC (TC*).
Once you have TC*, you can substitute this value into the isocost equation above (10L + 50,000,000/L = TC) and then solve for L* (rounded to the nearest whole number).
Going back to (1), we can substitute in L* and TC*, to get K*.